Abstract
The hypoplastic model for sands proposed by Wolffersdorff (Mech Cohes Frict Mater 1: 251–271, 1996) combined with the intergranular strain anisotropy by Fuentes and Triantafyllidis (Int J Numer Anal Meth Geomech 39: 1235–1254, 2015) is herein extended to account for cyclic mobility effects to allow for the simulation of liquefaction phenomena. The extension is based on the introduction of an additional state variable that permits the detection of cyclic mobility paths. The simulation capabilities of the model is compared with undrained triaxial tests of Karlsruhe fine sand. At the end, a finite element simulation of an offshore monopile embedded in sand, exposed to environmental forces from the Caribbean Sea, is constructed and analyzed.

















Similar content being viewed by others
Notes
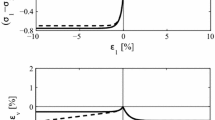
On the other hand, Wu and Niemunis [52] showed that the direction of tensor \(\mathbf {m}=-(\mathsf{L}^\mathrm{hyp})^{-1}:\mathbf {N}^\mathrm{hyp}\) coincides with the one of the accumulated strain under a closed infinitesimal stress cycle, which may be interpreted by some authors as a hypoplastic flow rule [11, 29, 52]. However, one may also show that its resulting dilatancy surface described by the condition \(m_{ii}=0\) coincides with the critical state surface, which does not depend on the void ratio and therefore disagrees with experiments, see “Appendix D”
References
Andrianopoulos K, Papadimitriou A, Bouckovalas G (2010) Bounding surface plasticity model for the seismic liquefaction analysis of geostructures. Soil Dyn Earthq Eng 30(10):895–911
API (American Petroleum Institute) (2014) Recommended practice for planning, designing and constructing fixed offshore platforms: working stress design. American Petroleum Institute, Washington
Arany L, Bhattacharya S, Macdonald J, Hogan SJ (2017) Design of monopiles for offshore wind turbines in 10 steps. Soil Dyn Earthq Eng 92:126–152
Boulanger R, Ziotopoulou K (2012) Pm4sand (version 2): a sand plasticity model for earthquake engineering applications. Technical report, University of California, Center for geotechnical modeling department of civil and environmental engineering, Davis, California, USA
Chang CS, Yin Z-Y (2010) Modeling stress-dilatancy for sand under compression and extension loading conditions. J Eng Mech 136(6):777–786
Dafalias Y, Manzari M (2004) Simple plasticity sand model accounting for fabric change effects. J Eng Mech ASCE 130(6):634–662
Das A, Bajpai P (2018) A hypo-plastic approach for evaluating railway ballast degradation. Acta Geotechnica 13(5):1085–1102
Devis-Morales A, Montoya-Sánchez RA, Bernal G, Osorio AF (2017) Assessment of extreme wind and waves in the Colombian Caribbean Sea for offshore applications. Appl Ocean Res 69:10–26
Fuentes W (2014) Contributions in mechanical modelling of fill materials. Schriftenreihe des Institutes für Bodenmechanik und Felsmechanik des Karlsruher Institut für Technologie, Heft 179
Fuentes W, Tafili M, Triantafyllidis T (2018) An isa-plasticity-based model for viscous and non-viscous clays. Acta Geotechnica 13(2):367–386
Fuentes W, Triantafyllidis T (2015) ISA model: A constitutive model for soils with yield surface in the intergranular strain space. Int J Numer Anal Meth Geomech 39(11):1235–1254
Fuentes W, Triantafyllidis T, Lascarro C (2017) Evaluating the performance of an isa-hypoplasticity constitutive model on problems with repetitive loading. In: Triantafyllidis T (ed) Holistic simulation of geotechnical installation processes, volume 82 lecture notes in applied and computational mechanics. Springer, Berlin, pp 341–362
Gasch R, Twele J (eds) (2012) Calculation of performance characteristics and partial load behaviour, chapter 6. Springer, Berlin, pp 208–256
Hansen MOL (2008) 1D momentum theory for an ideal wind turbine, chapter 4. Earthscan Publications Ltd, London, pp 27–40
Herle I, Kolymbas D (2004) Hypoplasticity for soils with low friction angles. Comput Geotech 31(5):365–373
Jonkman J, Butterfield S, Musial W, Scott G (February 2009) Definition of a 5-mw reference wind turbine for offshore system development. Technical report, National Renewable Energy Laboratory (NREL)
Kolymbas D (1991) Computer-aided design of constitutive laws. Int J Numer Anal Methods Geomech 8(15):593–604
Kolymbas D, Herle I (2005) Hypoplasticity as a constitutive framework for granular soils. In: ASCE, pp 257–289
Kuhn MR, Renken HE, Mixsell AD, Kramer SL (2014) Investigation of cyclic liquefaction with discrete element simulations. J Geotech Geoenviron Eng 140(12):04014075
Lade P, Ibsen L (1997) A study of the phase transformation and the characteristic lines of sand behaviour. geotechnical engineering group. Published In: Proceedings of international symposium on deformation and progressive failure in geomechanics, Nagoya, Japan, pp 353–359
LeBlanc C, Hededal O, Ibsen LB (2008) A modified critical state two-surface plasticity model for sand—theory and implementation. Technical report, Aalborg University, Department of Civil Engineering Water and Soil, Denmark
Li X, Dafalias Y (2000) Dilatancy for cohesionless soils. Géotechnique 50(4):449–460
Manwell JF, McGowan JG, Rogers AL (2009) Wind characteristics and resources, chapter 2. Wiley, Hoboken, pp 23–90
Masin D (2005) A hypoplastic constitutive model for clays. Int J Numer Anal Methods Geomech 29(4):311–336
Masin D, Herle I (2005) State boundary surface of a hypoplastic model for clays. Comput Geotech 32(6):400–410
Matsuoka H, Nakai T (1977) Stress-strain relationship of soil based on the SMP. In: Proceedings of speciality session 9, IX international conference on soil mechanics found Engineering, Tokyo, pp 153–162
Morison JR, Johnson JW, Schaaf SA (1950) The force exerted by surface waves on piles. J Pet Technol 2:149–154
Ng T, Dobry R (1994) Numerical simulations of monotonic and cyclic loading of granular soil. J Geotech Eng 120(2):388–403
Niemunis A (2003) Extended hypoplastic models for soils. Habilitation, Schriftenreihe des Institutes für Grundbau und Bodenmechanil der Ruhr-Universit”at Bochum, Germany, Heft 34
Niemunis A, Grandas C, Wichtmann T (2016) Peak stress obliquity in drained and undrained sands. Simulations with neohypoplasticity. Springer International Publishing, Cham, pp 85–114
Niemunis A, Herle I (1997) Hypoplastic model for cohesionless soils with elastic strain range. Mech Cohes Frict Mater 2(4):279–299
NRELs National Wind Technology Center (2018) Nrel 5-mw reference turbine - cp, cq, ct coefficients. https://wind.nrel.gov/forum/wind/viewtopic.php?f=2&t=582
Oka F, Kimoto S (2018) A cyclic elastoplastic constitutive model and effect of non-associativity on the response of liquefiable sandy soils. Acta Geotechnica 13(6):1283–1297
Osorio AF, Montoya RD, Ortiz JC, Peláez D (2016) Construction of synthetic ocean wave series along the colombian caribbean coast: a wave climate analysis. Appl Ocean Res 56:119–131
Poblete M, Fuentes W, Triantafyllidis T (2016) On the simulation of multidimensional cyclic loading with intergranular strain. Acta Geotechnica 11(6):1263–1285
Pradhan T, Tatsuoka F, Sato Y (1989) Experimental stress-dilatancy relations of sand subjected to cyclic loading. Soils Found 29(1):45–64
Sitharam TG, Dinesh SV (2003) Numerical simulation of liquefaction behaviour of granular materials using discrete element method. J Earth Syst Sci 112(3):479
Sitharam TG (2003) Discrete element modelling of cyclic behaviour of granular materials. Geotech Geol Eng 21(4):297–329
Taiebat M, Jeremic B, Dafalias Y, Kaynia A, Cheng Z (2010) Propagation of seismic waves through liquefied soils. Soil Dyn Earthq Eng 30:236–257
van der Tempel J (2006) Design of support structures for offshore wind turbines. PhD thesis, Delft University of Technology, Delft, Netherlands
Villalobos Jara FA (2006) Model testing of foundations for offshore wind turbines. PhD thesis, Oxford University, UK, United Kingdom
Vugts JH, van der Tempel J, Schrama EA (2001) Hydrodynamic loading on monotower support structures for preliminary design. In: Proceedings of special topic conference on offshore wind energy, Brussels, Belgium
Wang R, Fu P, Zhang J, Dafalias Y (2016) Dem study of fabric features governing undrained post-liquefaction shear deformation of sand. Acta Geotechnica 11(6):1321–1337
Wang S, Wei W, Peng C, He X, Cui D (2018) Numerical integration and FE implementation of a hypoplastic constitutive model. Acta Geotechnica 13(6):1265–1281
Wegener D, Herle I (2014) Prediction of permanent soil deformations due to cyclic shearing with a hypoplastic constitutive model. Geotechnik 37(2):113–122
Weifner T, Kolymbas D (2007) A hypoplastic model for clay and sand. Acta Geotechnica 2(2):103–112
Wichtmann T, Triantafyllidis T (2014) Stiffness and damping of clean quartz sand with various grain-size distribution curves. J Geotech Geoenviron Eng 140(3):1–4
Wichtmann T, Triantafyllidis T (2016) An experimental database for the development, calibration and verification of constitutive models for sand with focus to cyclic loading: part i–tests with monotonic loading and stress cycles. Acta Geotechnica 11(4):739–761
Wolffersdorff A (1996) A hypoplastic relation for granular materials with a predefined limit state surface. Mech Cohes Frict Mater 1(3):251–271
Wu W (1992) Hypoplastizität als mathematisches modell zum mechanischen Verhalten von Böden und Schöttstoffen. Phd thesis, Universität Karlsruhe, Germany. Institut für Boden- und Felsmechanik, Habilitation, Heft 129
Wu W, Bauer E (1994) A simple hypoplastic constitutive model for sand. International Journal for Numerical and Analytical Methods in Geomechanics 18(12):833–862
Wu W, Niemunis A (1996) Failure criterion, flow rule and dissipation function derived from hypoplasticity. Mechanics of Cohesive-Frictional Materials 1(2):145–163
Yin Z-Y, Chang CS (2011) Stress-dilatancy behavior for sand under loading and unloading conditions. International Journal for Numerical and Analytical Methods in Geomechanics 37(8):855–870
Acknowledgements
The authors appreciate the financial support given by COLCIENCIAS (Colombia) for the project with code 1215748-59323 from the convocation 748-2016, and the one given by the Bolivar Department (Colombia) and administered by CeiBA, through the scholarship “Bolivar wins with science”.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Notation and conventions
The notation and convention of the present work is as follows: Italic fonts denote scalar magnitudes (e.g., a, b), bold lowercase letters denote vectors (e.g., \(\mathbf {a}, \mathbf {b}\)), bold capital letters denote second-rank tensors (e.g., \(\mathbf {A}\), \({\varvec{\sigma }}\)), and special fonts are used for fourth-rank tensors (e.g., \(\mathsf{E}, \mathsf{L}\)). Indicial notation can be used to represent components of tensors (e.g., \(A_{ij}\)), and their operations follow the Einstein’s summation convention. The Kronecker delta symbol is represented by \(\delta _{ij}\), i.e., \(\delta _{ij}=1\) when \(i=j\) and \(\delta _{ij}=0\) otherwise. The symbol \(\mathbf {1}\) denotes the Kronecker delta tensor (\(1_{ij}=\delta _{ij}\)). The unit fourth-rank tensor for symmetric tensors is denoted by \(\mathsf{I}\), where \(\mathsf{I}_{ijkl}=\frac{1}{2}\left( \delta _{ik}\delta _{jl}\right.\)\(\left. +\delta _{il}\delta _{jk}\right)\). Multiplication with two dummy indices (double contraction) is denoted with a colon “ : ” (e.g., \(\mathbf {A}:\mathbf {B}=A_{ij}B_{ij}\)). The symbol “\(\otimes\)” represents the dyadic product (e.g., \(\mathbf {A}\otimes \mathbf {B}=A_{ij}B_{kl}\)). The brackets \(\parallel \bigsqcup \parallel\) extract the Euclidean norm (e.g., \(\parallel \mathbf {A}\parallel =\sqrt{A_{ij}A_{ij}}\)). Normalized tensors are denoted by \(\overrightarrow{\bigsqcup }=\frac{\bigsqcup }{\parallel \bigsqcup \parallel }\), or in general as \(\sqcup ^{\rightarrow }\). The superscript \(\bigsqcup ^\mathrm{dev}\) extracts the deviatoric part of a tensor (e.g., \(\mathbf {A}^\mathrm{dev}=\mathbf {A}-\frac{1}{3}(\mathrm {tr}\mathbf {A})\mathbf {1}\)). Components of the effective stress tensor \({\varvec{\sigma }}\) or strain tensor \({\varvec{\varepsilon }}\) in compression are negative. Roscoe variables are defined as \(p=-\sigma _{ii}/3\), \(q=\sqrt{\frac{3}{2}}\parallel {\varvec{\sigma }}^\mathrm{dev}\parallel\), \(\varepsilon _v=-\varepsilon _{ii}\) and \(\varepsilon _s=\sqrt{\frac{2}{3}}\parallel {\varvec{\varepsilon }}^\mathrm{dev}\parallel\). The stress ratio \(\eta\) is defined as \(\eta =q/p\). The deviator stress tensor is defined as \({\varvec{\sigma }}^\mathrm{dev}={\varvec{\sigma }}+p\,\mathbf {1}\) and the stress-ratio tensor with \(\mathbf {r}={\varvec{\sigma }}^\mathrm{dev}/p=\sqrt{\frac{2}{3}}\,\eta \,\overrightarrow{{{\varvec{\sigma }}^\mathrm{dev}}}\).
Empirical relation for shear degradation curve by Wichtmann and Triantafyllidis [47]
The empirical relation provided by Wichtmann and Triantafyllidis [47] is:
with the relative density \(D_r=(e_\mathrm{max}-e)/(e_\mathrm{max}-e_\mathrm{min})\) and the reference stress \(p_\mathrm{atm}=100\) kPa. For Karlsruhe fine sand \(e_\mathrm{max}=1.054\) and \(e_\mathrm{min}=0.677\). The secant shear modulus \(G_\mathrm{sec}\) is computed with the empirical relation provided by Wichtmann and Triantafyllidis [47] :
where \(\Delta \gamma\) is the shear strain amplitude, \(a=1.070\ln (c_u)\) is a constant, \(\gamma _r=\tau _\mathrm{max}/G_\mathrm{max}\) is the reference strain, \(\tau _\mathrm{max}=p\sin (\varphi _p)\) is the maximum shear stress and \(\varphi _p=34^\circ \exp (0.27D_r^{1.8})\) is the peak friction angle. \(c_u\) is the uniformity coefficient (\(c_u=D_{60}/D_{10}\)). For Karlsruhe fine sand, \(c_u=1.5\) and therefore \(a=0.433\).
For drained triaxial conditions, the strain amplitude \(\parallel \Delta {\varvec{\varepsilon }}\parallel\) is computed with the following approximation \(\parallel \Delta {\varvec{\varepsilon }}\parallel =\sqrt{(\Delta \varepsilon _1)^2(1+2\nu ^2)}\) where \(\nu\) is the Poisson ratio. For same conditions, it can be shown that the relation \(\Delta \gamma =\Delta \varepsilon _1(1+\nu )\) holds. For the computations with Karlsruhe fine sand, a value of \(\nu =0.3\) was used.
The resulting parameters for \(e_0=0.85\) and \(p=200\) kPa are \(\gamma _r=9.29\times 10^{-4}\), \(G_\mathrm{max}=130058\) kPa. For \(e_0=0.85\) and \(p=300\) kPa are \(\gamma _r=1.14\times 10^{-3}\) and \(G_\mathrm{max}=158002\) kPa.
ISA-hypoplastic model for sands
“Appendix C” presents a summary of the constitutive equations of the ISA-hypoplastic model. Details of the equations below are found in [11, 35, 49].
where \(\mathsf{L}^\mathrm{hyp}\) and \(\mathbf {N}^\mathrm{hyp}\) are the (fourth rank) linear and (second rank) nonlinear stiffness, respectively, \(\mathbf {N}=(\mathbf {h}-\mathbf {c})^{\rightarrow }\) is the IS flow rule, \(m_R\) is a parameter, and \(\rho\), m, \(\chi\) and \(F_H\) are scalar functions defined in the sequel. The IS yield surface function \(F_H\) is defined as:
where \(\mathbf {h}\) is the IS tensor, \(\mathbf {c}\) is the back-IS tensor, and R is a parameter. Factors m, \(y_h\) and \(\rho\) are defined as:
The evolution equation for the IS tensor \(\mathbf {h}\) is:
where \(\dot{\lambda }_H\) is the plastic multiplier of the IS model. The evolution equation for tensor \(\mathbf {c}\) is:
where \(\beta _h\) is a factor, which takes the value of \(\beta _h=\beta _\mathrm{hmax}\) for the condition \(\mid \overrightarrow{\mathbf {h}_b}:\overrightarrow{\mathbf {d}_b}\mid =0\), and \(\beta _h=\beta _{h0}\) for \(\mid \overrightarrow{\mathbf {h}_b}:\overrightarrow{\mathbf {d}_b}\mid =1\).
The internal variable \(\dot{\varepsilon }_\mathrm{acc}\) evolves according to:
Function \(\chi\) is defined as:
The equations of the reference hypoplastic model by Wolffersdorff [49] are given below:
The set of parameters are \(\varphi _c\), \(h_s\), \(n_B\), \(e_{i0}\), \(e_{c0}\), \(e_{d0}\), \(\alpha\), \(\beta\), R, \(\chi _0\), \(\chi _\mathrm{max}\), \(\beta _{h0}\), \(\beta _\mathrm{hmax}\), \(c_a\). The state variables are e, \(\mathbf {c}\), \(\mathbf {h}\) and \(\varepsilon _\mathrm{acc}\).
Inspection of hypoplastic flow rule tensor \(\mathbf {m}\)
Substitution of Eqs. 36 and 37 in tensor \(\mathbf {m}=-(\mathsf{L}^\mathrm{hyp})^{-1}:\mathbf {N}^\mathrm{hyp}\) gives (see procedure in [29]):
Splitting tensor \(\mathbf {m}\) into volumetric and deviatoric components \(\mathbf {m}^\mathrm{vol}=\mathsf{I}^\mathrm{vol}:\mathbf {m}\) and \(\mathbf {m}^\mathrm{dev}=\mathsf{I}^\mathrm{dev}:\mathbf {m}\), where \(I^\mathrm{vol}_{ijkl}=1/3\delta _{ij}\delta _{kl}\) and \(I^\mathrm{dev}_{ijkl}=I_{ijkl}-I^\mathrm{vol}_{ijkl}\) gives:
Where fuction \(f_\sigma\) is defined as:
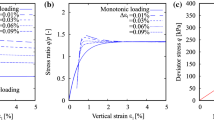
Simulations of monotonic loading
In this “Appendix”, some simulations of the Karlsruhe fine sand under monotonic loading are presented. Simulations versus experiments are shown in Figs. 18 and 19. Parameters of Table 1 were used for the simulations.
Rights and permissions
About this article
Cite this article
Fuentes, W., Wichtmann, T., Gil, M. et al. ISA-Hypoplasticity accounting for cyclic mobility effects for liquefaction analysis. Acta Geotech. 15, 1513–1531 (2020). https://doi.org/10.1007/s11440-019-00846-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11440-019-00846-2